Answer:
0.191 is the probability that a group of 12 randomly selected applicants would have a mean SAT score that is greater than 525 but below the current admission standard of 584.
Explanation:
We are given the following information in the question:
Mean, μ = 500
Standard Deviation, σ = 100
n = 12
We are given that the distribution of SAT score is a bell shaped distribution that is a normal distribution.
Formula:
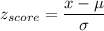
P(greater than 525 but 584)
Standard error due to sampling =
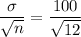
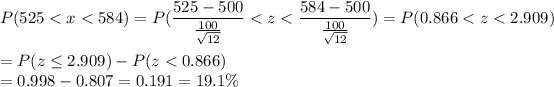
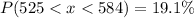
0.191 is the probability that a group of 12 randomly selected applicants would have a mean SAT score that is greater than 525 but below the current admission standard of 584.