To solve the problem it is necessary to take into account the concepts related to energy efficiency in the engines, the work done, the heat input in the systems, the exchange and loss of heat in the soupy the radius between the work done the lost heat ( efficiency).
By definition the efficiency of the heat engine is
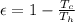
Where,
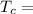 Temperature at the room
Temperature at the room
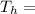 Temperature of the soup
Temperature of the soup
The work done is defined as,

Where
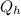 represents the input heat and at the same time is defined as
represents the input heat and at the same time is defined as
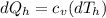
Where,
 Specific Heat
Specific Heat
The change at the work would be defined then as

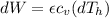
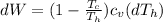
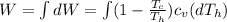
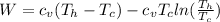
On the other hand we have that the heat lost by the soup is equal to
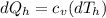
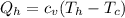
The ratio between both would be,
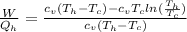
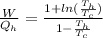
Replacing with our values we have,
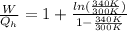
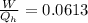
Therefore the fraction of heat lost by the soup that can be turned into useable work by the engine is 0.0613.