Answer:
C)
region C
Explanation:
We have to use what is called the zero-interval test [test point] in order to figure out which portion of the graph these inequalities share:
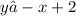
0 ≤ 2 ☑ [We shade the portion of the graph that CONTAIN THE ORIGIN, which is the bottom portion.]

0 ≥ −3 ☑ [We shade the portion of the graph that CONTAINS THE ORIGIN, which is the left side.]
So, now that we got that all cleared up, we can tell that both graphs share a region where the ORIGIN IS VISIBLE. Therefore region C matches the above inequalities.
I am joyous to assist you anytime.