Question

How many more unit tiles must be added to the function f(x)=x2−6x+1 in order to complete the square?

Answer 1

8 just took the test on edg

Explanation:

Answer 2

8 unit tiles should be added

Explanation:

Function given is
`f(x)=x^(2)-6x+1`. We wish to add more unit tiles to the function so that it becomes a complete square.

Since the given function is of order 2, the side of the square will be order 1. Let us assume a general order 1 expression
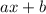`ax+b` to be the side of the square.

As the function forms the square after adding some
`p` unit tiles,
`f(x)+p=(\text{Side of square})^(2)`

`x^(2)-6x+1+p=(ax+b)^(2)=a^(2)x^(2) +2abx+b^(2)`

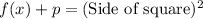
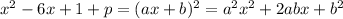
From comparision,
`a^(2)=1; 2ab=-6; b^(2)=1+p\\a=1; b=-3\\(-3)^(2)=p+1\\p=8`

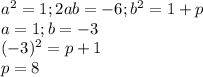
∴ 8 more unit tiles are required to complete the square.