Question

A Carnot heat engine receives heat from a heat source at 1200°C and has a thermal efficiency of 40 percent. The heat engine does maximum work equal to 490 kJ. Determine the heat supplied to the heat engine by the heat source, the heat rejected to the heat sink, and the temperature of the heat sink. (Round the final answers to the nearest whole number.)

Answer 1

Answered

Step-by-step explanation:

Given

Heat source temperature T_1= 1200° C = 1473 K

thermal efficiency η =40% = 0.4

maximum work output W_{max} = 490 kJ

we know that

`\eta= (W_(max))/(Q_1)`

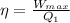
Heat supplied Q_1 can be calculated as 1225 kJ by putting values of η and W_{max} in the above equation.

Now we also know that
`W_(max)= Q_1-Q_2`

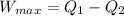
where Q_2 is the heat rejected to the sink

now
`Q_2= Q_1-W_(max)`

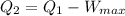
therefore, Q_2= 1225-490= 735 kJ

to calculate sink temperature T_2

we use the formula

`\eta= 1-(T_2)/(T_1)`

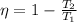
putting values of η and T_1 we get

T_2= 883.8 K= 610.8° C

Answer 2

T₂=610.8⁰C

Qa=1225 KJ

Qr=735 KJ

Step-by-step explanation:

Given that

T₁ = 1200⁰C

T₁ =1473 K

η= 40% = 0.4

We know that efficiency of Carnot cycle given as

`\eta=1-(T_2)/(T_1)`

T₁ = temperature of source

T₂= temperature of sink

`\eta=1-(T_2)/(T_1)`

`0.4=1-(T_2)/(1473)`

T₂=883.8 K

T₂=610.8⁰C

Lets take heat supplied is Qa

We know that

`\eta=(W)/(Q_a)`

W= Net work output

`\eta=(W)/(Q_a)`

`0.4=(490)/(Q_a)`

Qa=1225 KJ

From first law of thermodynamics

Qa= W+ Qr

Qr=Heat rejected to sink

Qa= W+ Qr

1225 = 490 + Qr

Qr=735 KJ