Question

The center of a hyperbola is (−4,3) , and one vertex is (−4,7) . The slope of one of the asymptotes is 2.

What is the equation of the hyperbola in standard form?

Answer 1

`((x+4)^(2))/(4) - ((y-3)^(2))/(16) = 1`

Explanation:

The hyperbola centered at (h,k) has the following expression:

`((x-h)^(2))/(a^(2)) - ((y-k)^(2))/(b^(2)) = 1`

Where
`a` and
`b` are the length of the horizontal and vertical semi-axes, respectively.

Since the center and one vertex share the same vertical component (
`x=-4`), it is easy to conclude that hyperbola has a vertical configuration (
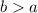`b > a`). The distance between the center and the known vertex is equal to the length of the vertical semi-axis. Therefore:

`b = 4`

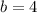
The slope of the hyperbola is given by the following relationship:

`(b)/(a) = 2`

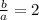
The length of the horizontal semi-axis is:

`a = (b)/(2)`

`a = 2`

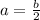
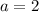
The standard form of the equation of the hyperbola is:

`((x+4)^(2))/(4) - ((y-3)^(2))/(16) = 1`

Answer 2

The answer to your question is below

Explanation:

C (-4, 3)

V (-4, 7)

asymptotes = 2 =
`(b)/(a)`

- This is a vertical hyperbola, the equation is

`((y - k)^(2) )/(a^(2) ) + ((x - h)^(2) )/(b^(2) ) = 1`

slope = 2

a is the distance from the center to the vertex = 4

b = 2(4) = 8

`((y - 3)^(2) )/(4^(2) ) + ((x + 4)^(2) )/(8^(2) ) = 1`

`((y - 3)^(2) )/(16) + ((x + 4)^(2) )/(64) = 1`