Question

In Triangle XYZ, A is the midpoint of XY, B is the midpoint of YZ, and C is the midpoint of XZ.

Also, AY = 7, BZ = 8, and XZ = 18. What is the perimeter of Triangle ABC? Explain

Answer 1

24

Explanation:

We have the theorem that if we join the midpoints of two sides of a triangle then the line so formed will be half of the third side of the triangle.

So, here in this case in triangle XYZ, A is the mid point of XY and B is the mid point of YZ,

Hence, Segment AB = Half of segment XZ =
`(18)/(2) =9`

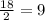
Similarly, Segment BC = Half of segment XY = Segment AY =7

{Since, Segment AY = Half of segment XY}

And again, Segment CA = Half of segment YZ = Segment BZ = 8

So, the perimeter of triangle ABC is = AB + BC + CA = 9 + 7 + 8 = 24. (Answer)