Answer:
x = 4, y = 6, z = 7
Explanation:
Given that for a triangle FGH, lines s, t and u are perpendicular bisectors.
They concur at the point J.
We know that the point of concurrence of perpendicular bisectors is the circmcenttre of the triangle. Hence we will have J equidistant from the three vertices. In other words,
JG = JH=JF
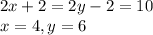
Now to find Z we make use of HI.

So option a is right answer
x = 4, y = 6, z = 7