Question

Breathing air that contains 4.0% by volume CO2 over time causes rapid breathing, throbbing headache, and nausea, among other symptoms. You may want to reference (Pages 539 - 541) Section 13.4 while completing this problem. Part A What is the concentration of CO2 in such air in terms of molarity, assuming 1 atm pressure and a body temperature of 37 ∘C? Express you

Answer 1

`1.6x10^(-4)M`

Step-by-step explanation:

Hello,

By volume percentage for this case is defined as:

`v/v=(V_(CO_2))/(V)*100`

Since we've got a 4.0%by volume solution, it is related, for instance, with 4L of carbon dioxide per 100L of air, so the moles are computed via the ideal gas equation as:

`n_(CO_2)=(PV)/(RT)=(1atm*4L)/(0.082(atm*L)/(mol*K)*310K)  \\n_(CO_2)=0.16molCO_2`

Finally, applying the molarity formula we get:

`M=(n_(CO_2))/(V) =(0.16mol)/(100L)=1.6x10^(-4)M`

Best regards.

Answer 2

Answer: The concentration of carbon dioxide in air is
`1.57* 10^(-3)M`

Step-by-step explanation:

We are given:

4.0 % carbon dioxide by volume

This means that 4.00 mL of carbon dioxide is present in 100 mL of solution

To calculate the amount of carbon dioxide, we use the equation given by ideal gas which follows:

`PV=nRT`

where,

P = pressure of the gas = 1 atm

V = Volume of the gas = 4.0 mL = 0.004 L

T = Temperature of the gas =
`37^oC=[37+273]K=310K`

R = Gas constant =
`0.0821\text{ L atm }mol^(-1)K^(-1)`

n = number of moles of carbon dioxide gas = ?

Putting values in above equation, we get:

`1atm* 0.004L=n* 0.0821\text{ L. atm }mol^(-1)K^(-1)* 310K\\\\n=(1* 0.004)/(0.0821* 310)=1.57* 10^(-4)mol`

To calculate the molarity of solution, we use the equation:

`\text{Molarity}=\frac{\text{Number of moles}* 1000}{\text{Volume of solution( in mL)}}`

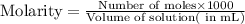
Moles of carbon dioxide gas =
`1.57* 10^(-4)mol`

Volume of solution = 100 mL

Putting values in above equation, we get:

`\text{Molarity of carbon dioxide}=(1.57* 10^(-4)* 1000)/(100)\\\\\text{Molarity of carbon dioxide}=1.57* 10^(-3)M`

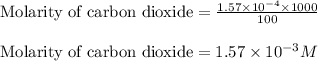
Hence, the concentration of carbon dioxide in air is
`1.57* 10^(-3)M`