Question

An electrical utility delivers 6.25E10 kWh of power to its customers in a year. What is the average power required during the year? If the powerplants supplying the utility have an average efficiency of 45% how much energy (in quads) does the power plants use in a year? Assuming that the power plants burn natural gas how many cubic feet of natural gas will be required to generate that much power?

Answer 1

The overall Utility delivered to customers in a year 'U' = 6.25 X 10¹⁰Kwh

However, the average power P, required for a year, t = ? Kw

Expressing their relationship, we will have

U = P x t

Given t = 1 year = 24 x 365 hours (assume a year operation is 365 days)

t = 8760 hours

P =
`(62500000000)/(8760)`

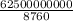
P = 7134.7Kw

Hence, the average power required during the year is 7,135Kw

Now to calculate the energy used by the power plant in a year (in quads)?

Recall, Efficiency, η = Power Output/Power Input (100)

so, we have

η = P₀/P₁, given

0.45 =
`(7134.7Kw)/(P₁)`

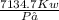
P₁ = 15,855Kw

the total energy E₁ used in a year = 15,855x24x365 = 138.89MJoules

So to convert this to quads, Note;

1 quads of energy = 10¹⁵ Joules

The total energy used is 0.000000139 quads

Now to find the cubic feet of natural gas required to generate this power?

Note: 0.29Kwh of Power generated = 1 cubic feet of natural gas used

Since, the power plant generated = 62500000000Kwh

The cubic feet of natural gas used =
`(62500000000)/(0.29)`

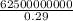
Hence, 2.155x10²⁰cubic feet of N.gas was used to generate this much power.