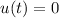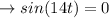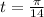Question

g A mass of 100 g stretches a spring 5 cm. If the mass is set in motion from its equilibrium position with a downward velocity of 30 cm/s, and if there is no damping, determine the position u of the mass at any time t. (Use g = 9.8 m/s2 for the acceleration due to gravity. Let u(t), measured positive downward, denote the displacement in meters of the mass from its equilibrium position at time t seconds.)

Answer 1

This is a Physics problem linked to differential equations.

In order to solve it, we define our initial values:

`m=0.1Kg\\x=0.05m\\g=9.8m/s^2`

We have given initial conditions,

The mass starts from a point of displacement at rest, that is,

`U(0)=0`

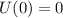
However, it has a resting point speed equal to 30cm/s

`U'(0) = 0.3m/s`

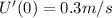
By definition we know that,

By Newton's law we know that

`F=mg`

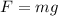
And by Hook's law we know that

`F=kX`

where,

k elongation / elastic constant

X the change or distance traveled

Equating the expressions we have

`mg=kX`

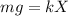
Solving for k,

`k=(mg)/(x)`

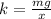
Replacing,

`k= ((0.1)(9.8))/(0.05)`

`k=19.6N/m`

The differential equation given for the movement is,

`mu(t)''+ku(t)=0`

`0.1 u(t)''+19.6u(t)=0`

`u(t)''+196u(t)=0`

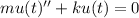
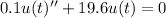
Being a differential equation we can obtain its auxiliary equation, given by

`r^2+196=0`

`r= \pm 14i`

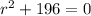
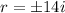
By definition, this is a second order linear differential equation,

We know that every function of the form

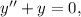`y''+y=0,` its solution is

`y(t)=c*cos(t)+d*sin(t)`

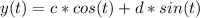
Applying the definition,

`u(t)=c*cos(14t)+dsin(14t)`

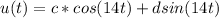
And the first derivative would be

`u'(t)=14c*cos(14t)-14dsin(14t)`

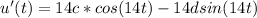
Applying our ideal conditions we can find d and c, so

For u(0) =0

`u(0) = c*cos(14*0)+sin(14*0)`

`c=0`

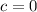
For u(0)=0.3

`u'(0)=14c*cos(14*0)-14dsin(14*0)`

`u'(0)=3/140`

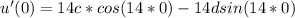
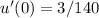
We have know that our equation for the position of the mass at any time is,

`u(t)=(3)/(140)sin(14t)`

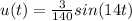
Solving for the question: The time when the mass return to equilibrium we have

`u(t)=0`

`0=(3)/(140)sin(14t)`

`\rightarrow sin(14t)=0`

`t=(\pi)/(14)`