Question

Suppose that engineering specifications on the shelf depth of a certain slug to be turned on a CNC lathe are from .0275 in. to .0278 in. and that values of this dimension produced on the lathe can be described using a normal distribution with mean μ and standard deviation σ . (a) If μ = .0276 and σ = .0001, about what frac- tion of shelf depths are in specifications? (b) What machine precision (as measured by σ ) would be required in order to produce about 98% of shelf depths within engineering spec- ifications (assuming that μ is at the midpoint of the specifications)?

Answer 1

0.8188 or 82%

0.0000643

Explanation:

Given that engineering specifications on the shelf depth of a certain slug to be turned on a CNC lathe are from .0275 in. to .0278 in.

If x is the dimension then X is N(0.0276, 0.0001)

Or
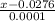`(x-0.0276)/(0.0001)` is Normal (0,1)

a) Fraction of shelf depths are in specifications

=
`P(0.0275<X<0.0278)\\=P(-1<z<2)\\= 0.3413+0.4775\\= 0.8188`

b) For 98% to be within the specifications,

`\mu = 0.02765`

Margin of error =
`0.0278-0.02775\\=0.00015`

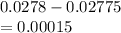
For 98% critical value Z two tailed is 2.33

Hence std error =
`(0.00015)/(2.33) \\=0.0000643`

So sigma should be = 0.0000643