Question

It takes George 1 hour longer to mow the lawn than it takes Henry. Working together, using two mowers, they can mow the lawn in 1 hour and 12 minutes. How long would it take Henry to mow the lawn by himself? [hint: Let x be the time taken by George. How much of the lawn does George mow in 1 hour? How much does Henry do in 1 hour? How much do they mow together in 1 hour?]

Answer 1

Explanation:

Given

George take 1 hr longer than Henry

Let Henry take x hr to complete the work

Therefore time taken by George is x+1 hr

Rate of George is
`(1)/(x+1) \hr`

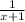
Rate of Henry is
`(1)/(x) \hr`

They both complete the work in 1 hr and 12 min i.e
`(6)/(5) hr`

therefore they both mow in hr

`(1)/(x+1)+(1)/(x)=(5)/(6)`

`(x+x+1)/((x+1)(x))=(5)/(6)`

`5x^2-7x-6=0`

`(x-2)(5x+3)=0`

`x=2 hr`

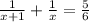
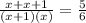
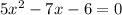
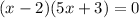
thus George mow
`(1)/(3)` lawn in 1 hr

Henry mow
`(1)/(2)` lawn in 1 hr

both mow
`(5)/(6)` in 1 hr