Answer:
Explanation:
Given that we can Assume the results come from random samples, and if the sample sizes are small, assume the underlying distribution of the differences is relatively normal. Assume that differences are computed using d=x1-x2. Test H0 : μd=0 vs Ha : μd≠0
Here test statistic = Mean difference ./std error
= Mean difference/(std dev/(sqrt n))
=
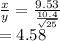
df =24
p value = 0.000121
Since p < alpha, we reject null hypothesis.
The two means are significantly different.