Answer:
All whole numbers are integers.
All natural numbers are rational numbers.
All irrational numbers are dense.
Explanation:
1) All real numbers are natural numbers. - WRONG.
Consider
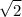 . This is a real number but a natural number which consists of integers
. This is a real number but a natural number which consists of integers
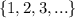 .
.
2) All whole numbers are integers. - CORRECT.
Whole numbers consist of natural numbers and zero. i.e.,
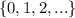 . All these numbers are integers. Hence the statement is correct.
. All these numbers are integers. Hence the statement is correct.
3) All integers are whole numbers. - WRONG.
Consider
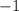 . This is an integer. But not a whole number.
. This is an integer. But not a whole number.
4) All natural numbers are rational numbers - CORRECT.
All natural numbers are a subset of rational numbers.i.e., rational numbers with denominator
 , In fact they are a subset of real numbers as well. Hence the statement is correct.
, In fact they are a subset of real numbers as well. Hence the statement is correct.
Note that the converse isn't true,
5) All irrational numbers are dense - CORRECT.
All irrational and rational numbers are dense on a real line. This basically means that for any two irrational numbers on a real line there is always another irrational number between them. This holds for true for rational numbers as well.