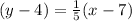Question

Complete the slope equation of the line through 2,3 and 7, 4

Y-4=

Answer 1

The slope equation of the line AB is
`(y -4) = (1)/(5) (x-7)`

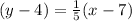
Explanation:

Here, the given points are A (2, 3) and B (7,4).

Now, slope of any line is given as :

`m = (y_2 - y_1)/(x_2 - x_1)`

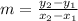
or,
`m = (4 -3)/(7-2)   = (1)/(5)`

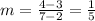
Hence, the slope of the line AB is (1/5)

Now , A POINT SLOPE FORM of an equation is

(y - y0) = m (x - x0) ; (x0, y0) is any arbitrary point on line.

Hence, the equation of line AB with slope (1/5 ) and point (7,4) is given as:

`(y  -4 ) = (1)/(5)  ( x - 7)`

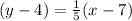
or, 5y - 20 = x -7

⇒ x - 57 + 13 = 0 ( SIMPLIFIED FORM of equation)

Hence, the slope equation of the line AB is
`(y -4) = (1)/(5) (x-7)`