Question

Guadalupe and her three year old son Carlos visited the pediatrician so carlos could get a check up. the doctor told guadalupe that his height was one standard deviation above the mean height for all three year olds. if the height for three year old boys is normally distributed with a mean of 37 inches and a standard deviation of 1 inch, how tall is carlos? note that you solve for x (his height) using the mean and standard deviation.

Answer 1

Carlos' height is 38 inches.

Explanation:

Problems of normally distributed samples can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

In this problem, we have that:

The height for three year old boys is normally distributed with a mean of 37 inches and a standard deviation of 1 inch. This means that
`\mu = 37, \sigma = 1`.

The doctor told guadalupe that his height was one standard deviation above the mean height for all three year olds. How tall is Carlos?

Since his height is one standard deviation above the mean, we have that
`Z = 1`.

Carlos' height is X.

`Z = (X - \mu)/(\sigma)`

`1 = (X - 37)/(1)`

`X - 37 = 1`

`X = 38`

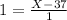
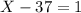
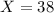
Carlos' height is 38 inches.