Question

If f(x) is discontinuous, determine the reason. f(x)= {x^2 +4, x<=1; x+3, x>1}

Answer 1

Observe that f(x) is a continuous function when
`x<1\; and\; x>1` because is a polynomial. The possible problem may occur in x=1.

Then, f(x) is discontinuous in x=1 if the limits of f to the right and the left of 1 exist and are different or if some of those limits doesn't exist.

Let's calculate the limits:

`lim_(x\rightarrow 1^+)f(x)=lim_(x\rightarrow 1)(x+3)=1+3=4`

`lim_(x\rightarrow 1^-)f(x)=lim_(x\rightarrow 1^-)(x^2+4)=1^2+4=5`

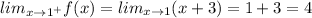
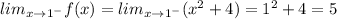
Since,
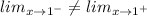`lim_(x\rightarrow 1^-)\\eq lim_(x\rightarrow 1^+)` then f(x) is discontinuous in x=1.