Question

In circle O, AC and BD are diameters. Circle O is shown. Line segments A C and B D are diameters. The measure of arc A B is (3 x minus 70) degrees and the measure of arc D C is (x + 10) degrees. What is mArc B C? 50° 80° 100° 130°

Answer 1

130

Explanation:

Answer 2

The measurement of arc BC is 130°.

Explanation:

Given : circle with origin O, with two line segments AC and BD which are also diameters.

Measurement of arc AB = (3 x -70)° =

Measurement of arc DC= (x +10)° = ∠3

To find : Measurement of arc BC = ∠2 =?

Solution:

Let angle subtended by the arc AC = ∠1 = (3 x -70)°

Let angle subtended by the arc BD = ∠2

Let angle subtended by the arc DC = ∠3 = ( x + 10)°

Let angle subtended by the arc AD = ∠4

AC and BD are intersecting each other at O.

So, ∠1 = ∠3 (vertically opposite angles)..[1]

∠2 = ∠4 (vertically opposite angles)..[2]

(3 x -70)° = (x +10)° (from [1])

3x - x = 10 + 70

`x=(10 + 70)/(2)=40`

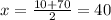
∠1 = ∠3 = (x +10)° = (40 +10)° = 50°

∠1 + ∠2 + ∠3 + ∠4 = 360°

50° + ∠2 + 50° + ∠2= 360° (from [2])

2∠2 = 360° -50° - 50°

2∠2 = 260°

∠2 = 260° ÷ 2

∠2 = 130°

The measurement of arc BC is 130°.