a. 661.23 m/s
b. the rate of effusion of Ammonia = 4.5 faster than Silicon tetra bromide
Further explanation
Given
T = 25 + 273 = 298 K
Required
a. the gas speed
b. The rate of effusion comparison
Solution
a.
Average velocities of gases can be expressed as root-mean-square averages. (V rms)
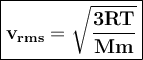
R = gas constant, T = temperature, Mm = molar mass of the gas particles
From the question
R = 8,314 J / mol K
T = temperature
Mm = molar mass, kg / mol
Molar mass of Ammonia = 17 g/mol = 0.017 kg/mol
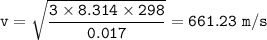
b. the effusion rates of two gases = the square root of the inverse of their molar masses:
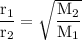
M₁ = molar mass Ammonia NH₃= 17
M₂ = molar mass Silicon tetra bromide SiBr₄= 348
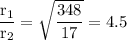
the rate of effusion of Ammonia = 4.5 faster than Silicon tetra bromide