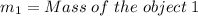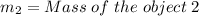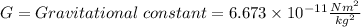Answer:
It depends on their masses, and the distance between them.
Step-by-step explanation:
According to Newton's Law of Universal Gravitation, every particle in the universe attracts any other particle with a strength that is directly proportional to the product of its masses and inversely proportional to the square of the distance between them. Mathematically it is expressed as:
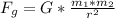
Where: