Question

Find the critical points of the function and use the First Derivative Test to determine whether the critical point is a local minimum or maximum (or neither). (Enter your answers as a comma-separated list. If an answer does not exist, enter DNE.) f(x) = 3 tan−1(x) − 3/2x + 5, on (−[infinity], [infinity])

Answer 1

Critical points are 1 and -1

Maximum at x=1

Minimum at x=-1

Explanation:

We are given that a function

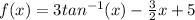`f(x)=3tan^(-1)(x)-(3)/(2) x+5` on (
`-\infty,\infty`)

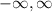We have to find the critical points of the function.

To find the critical point we will differentiate function w.r.t x and then substitute f'(x)=0

`f'(x)=(3)/(1+x^2)-(3)/(2)`

`(d(tan^(-1)x))/(dx)=(1)/(1+x^2)`

`f'(x)=0`

`(3)/(1+x^2)-(3)/(2)=0`

`(3)/(1+x^2)=(3)/(2)`

`1+x^2=2`

`x^2=2-1=1`

`x=\pm1`

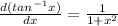
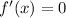
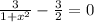
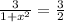
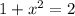
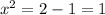
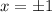
Therefore, the critical points of the given function are 1 and -1.

`f(0)=3-(3)/(2)=(3)/(2)`

`f'(1)=0`

`f'(2)=(3)/(5)-(3)/(2)=-(9)/(10)`

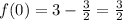
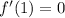
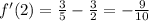
When we goes from 0 to 2 then the sign of derivative change from positive to negative .Therefore, function has local maximum at x=1.

`f(-2)=(3)/(5)-(3)/(2)=-(9)/(10)`

`f(-1)=0`

`f(0)=(3)/(2)`

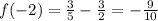
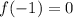
When we goes form -2 to 0 then the sign of derivative change from negative to positive .Hence , function has local minimum at x=-1

Hence, critical points are local maximum and local minimum .