Answer:
She jogged uphill for 1.6 miles
Explanation:
Given:
Uphill speed = 3 miles per hour
Downhill speed = 8 miles per hour
Total time to cover uphill and downhill = 40 minutes =
 hours
hours
Average speed = 4 miles per hour
Now,
Let the distance of uphill be 'x'
and downhill distance be 'y'
Time =
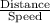
therefore,
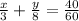
or
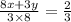
or
8x + 3y = 16 ...........(1)
also,
Average speed =
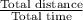
or
4 =

or
4 × 40 = 60(x + y)
or
3x + 3y = 8
or
3y = 8 - 3x ............(2)
substituting 2 in 1, we get
8x + (8 - 3x) = 16
or
5x + 8 = 16
or
5x = 8
or
x = 1.6 miles
and,
3y = 8 - 3x
or
3y = 8 - 3(1.6)
or
3y = 8 - 4.8
or
y = 1.067 miles
Hence,
She jogged uphill for 1.6 miles