Question

In an effort to estimate the mean of amount spent per customer for dinner at a major Lawrence restaurant, data were collected for a sample of 49 customers. Assume a population standard deviation of $5. If the sample mean is $24.80, what is the 99% confidence interval for the population mean?

Answer 1

The 99% confidence interval for the population mean is 22.96 to 26.64

Explanation:

Consider the provided information,

A sample of 49 customers. Assume a population standard deviation of $5. If the sample mean is $24.80,

The confidence interval if 99%.

Thus, 1-α=0.99

α=0.01

Now we need to determine
`z_{(\alpha)/(2)}=z_(0.005)`

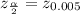
Now by using z score table we find that
`z_{(\alpha)/(2)}=2.58`

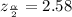
The boundaries of the confidence interval are:

`\mu-z_{(\alpha)/(2)}* (\sigma)/(√(n) )\\24.80-2.58* (5)/(√(49))=22.96\\\mu+z_{(\alpha)/(2)}* (\sigma)/(√(n) )\\24.80+2.58* (5)/(√(49))=26.64`

Hence, the 99% confidence interval for the population mean is 22.96 to 26.64