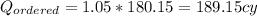Answer:
189.15cy
Step-by-step explanation:
To understand this problem we need to understand as well the form.
It is clear that there is four wall, two short and two long.
The two long are
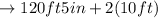
The two long are
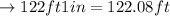
The two shors are

The height and the thickness are 14ft and 0.83ft respectively.
So we only calculate the Quantity of concrete,
![Q_c = [(2*122.08)+(2*86-375)]*14*0.833\\Q_c=4864.02ft^3](https://img.qammunity.org/2020/formulas/engineering/college/daow8qyg1tkev2d3ku8dnh1384p99s43ru.png)
That in cubic yards is equal to
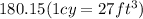
Hence, we need order 5% plus that represent with the quantity