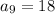Question

Write an expression for the sequence in

each table.
4.
Position
1 2 3 4 5 1
Value of Term 9
10 11 12 13

Answer 1

• 
  `a_n=n+9`

• 
  `a_9=18`

Explanation:

Determining the expression for the sequence:

Given the sequence

10, 11, 12, 13

Here, the first element is:

a₁ = 10

An arithmetic sequence has a constant difference 'd' and is defined by

`a_n=a_1+\left(n-1\right)d`

computing the differences of all the adjacent terms

`11-10=1,\:\quad \:12-11=1,\:\quad \:13-12=1`

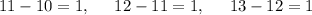
The difference between all the adjacent terms is the same and equal to

`d=1`

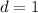
now substituting d = 1 and a₁ = 10 in the nth term

`a_n=a_1+\left(n-1\right)d`

`a_n=\left(n-1\right)+10`

`a_n=n+9`

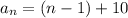
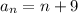
Determining the value of the 9th term

Given the nth term

`a_n=n+9`

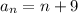
substituting n = 9 to determine the 9th term

`a_9=9+9`

`a_9=18`

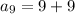
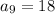
Therefore, the vale of the 9th term is:

`a_9=18`