Answer:
a) 2,093
b) It will reorder once there are 420 units left (demand during lead-time)
c) 34 days
Step-by-step explanation:
a) economic order quantity
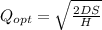
Where:
D = annual demand = 21,900
S= setup cost = ordering cost = 50
H= Holding Cost = 0.50

EOQ = 2092.844954
b) it takes four days to arrive:
if it sale 420 units per week then:
420 x 4/7 = 240 units are demand during delivery
c) order cycle:
EOQ / Annual Demand
2,093 / 21,900 = 0,09557 x 365 = 34.8333 days
It will order every 34 days (if it orders after 35 days will face shortage)