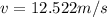Question

Lloyd is standing on a scaffolding 12 meters above the ground to clean the windows of a tall building. His bucket, which has a mass of 0.5 kilograms, falls off the edge of the scaffolding. Calculate the bucket’s kinetic and potential energy when it is 4 meters above the ground. Also calculate its velocity at this point.

Answer 1

U₂ = 20 J

KE₂ = 40 J

v= 12.64 m/s

Explanation:

Given that

H= 12 m

m = 0.5 kg

h= 4 m

The potential energy at position 1

U₁ = m g H

U₁ = 0.5 x 10 x 12 ( take g= 10 m/s²)

U₁ = 60 J

The potential energy at position 2

U₂ = m g h

U ₂= 0.5 x 10 x 4 ( take g= 10 m/s²)

U₂ = 20 J

The kinetic energy at position 1

KE= 0

The kinetic energy at position 2

KE= 1/2 m V²

From energy conservation

U₁+KE₁=U₂+KE₂

By putting the values

60 - 20 = KE₂

KE₂ = 40 J

lets take final velocity is v m/s

KE₂= 1/2 m v²

By putting the values

40 = 1/2 x 0.5 x v²

160 = v²

v= 12.64 m/s

Answer 2

The bucket's velocity is
`v = 12.522 m/s`

Explanation:

Given

Height above the ground, H = 12 m

Mass of bucket, m = 0.5 kg

Height of bucket, h = 4m

Taking acceleration of gravity, g as 9.8m/s²

First, we calculate the potential energy when bucket is on scaffolding (just about to fall) its total energy is 100%

P.E = mgH

P.E = 0.5 * 9.8 * 12

P.E = 58.8 J

At this point, the object is at rest, so the kinetic energy is 0 J

When the object is at 4 m above the ground, the bucket's total mechanical energy is as follows using conservation of energy

M.E
`= mgh + (1)/(2) mv^(2)`

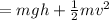
Where Total mechanical energy (M.E) = P.E (calculated above) = 58.8 J

By substitution, we have

`58.8 = 0.5 * 9.8 * 4 + (1)/(2) * 0.5 * v^(2)`

`58.8 = 19.6 + (1)/(2) * 0.5 * v^(2)`

`58.8 - 19.6 = (1)/(2) * 0.5 * v^(2)`

`39.2 = (1)/(2) * 0.5 * v^(2)`

`39.2 = (1)/(2) * (1)/(2) * v^(2)`

`39.2 * 2 * 2= v^(2)`

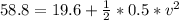
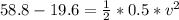
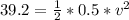
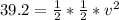
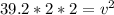
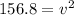`156.8= v^(2)` --- Take Square roots

`√(156.8) = \sqrt{v^(2)}`

`v = √(156.8)`

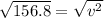
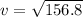
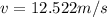`v = 12.522 m/s` ---- Approximated

Hence, the bucket's velocity is
`v = 12.522 m/s`