Question

Two blocks with different mass are attached to either end of a light rope that passes over a light, frictionless pulley that is suspended from the ceiling. The masses are released from rest, and the more massive one starts to descend. After this block has descended a distance 1.30 m, its speed is 3.50m/s . If the total mass of the two blocks is 14.0 kg, what is the mass of the more massive block?

Answer 1

m₁ = 10.36 Kg

Step-by-step explanation:

Newton's second law:

∑F = m*a Formula (1)

∑F : algebraic sum of the forces in Newton (N)

m : mass in kilograms (kg)

a : acceleration in meters over second square (m/s²)

Calculating of the acceleration (a)

Because the masses moves with uniformly accelerated movement we apply the following formula:

vf²=v₀²+2*a*d Formula (2)

Where:

d:displacement in meters (m)

vi: initial speed in m/s

vf: final speed in m/s

a: acceleration in m/s²

Data:

vi = 0

vf = 3.5 m/s

d = 1.3 m

We replace data in the formula (2) to calculate the acceleration of the masses:

vf²=vi²+2*a*d

`a=(v_(f)^(2)-v_(i) ^(2)   )/(2*d)`

`a= ((3.5)^(2) -0)/(2*1.3)`

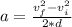
a= 4.7 m/s²

Problem development

m₁+m₂= 14.0 kg

g=9.81 m/s² acceleration due to gravity

W= m*g: Weight

• We take m₁> m₂

• We identify a direction as positive and observe that m₁ will accelerate downwards and m₂ will accelerate upwards, since m₁> m₂.

Newton's second law for m₁:

We apply the formula (1)

We take + in the direction of the downward movement:

∑F = m*a

W₁ -T = m₁ *a

W₁- m₁ *a=T Equation (1)

Newton's second law for m₂:

We take + in the direction of the upward movement:

∑F = m*a

T-W₂ = m₂*a

T = W₂+m₂*a Equation (2)

Equation (1)=Equation (2) =T

W₁- m₁ *a = W₂+m₂*a

W₁- W₂ = m₁ *a + m₂*a

(m₁-m₂ )*g =a*( m₁ + m₂ ) m₁+ m₂ =14 , m₂ =14-m₁

(m₁-(14-m₁ )*9.8 = 4.7(14)

(m₁-14+m₁ )= ( 4.7((14) / (9.8)

2m₁ = 6.71 + 14

2m₁ = 20.71

m₁ = 10.36 Kg

m₂= 14 kg-10.36 kg

m₂= 3.64 kg