Question

You toss 4 coins and if you toss all heads you win $9. If you toss anything other than 4 heads you lose $1. What is the Expected Value of the game?

Answer 1

The expected value of game is $
`(-3)/(8)`

Solution:

Given, You toss 4 coins and if you toss all heads you win $9.

When you toss anything other than 4 heads you lose $1

We have to find what is the Expected Value of the game?

The expected value is given as:

`\text { expected value }=\text { probability of winning } * \text { amount won }+\text { probability of losing } * \text { amount lost. }`

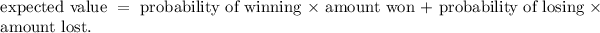
since we toss 4 coins, total outcomes
`= 2 * 2 * 2 * 2`

`\text {Expected value}=(1)/(2 * 2 * 2 * 2) * 9+(2 * 2 * 2 * 2-1)/(2 * 2 * 2 * 2) *(-1)`

`\begin{array}{l}{\text { Expected value }=(1)/(16) * 9+(16-1)/(16)(-1)} \\\\ {=(9)/(16)+(15)/(16)(-1)} \\\\ {=(9)/(16)-(15)/(16)=(-6)/(16)=(-3)/(8)}\end{array}`

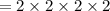
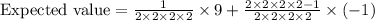
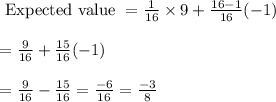
Hence, the expected value is
`(-3)/(8)`