Answer:
D.
Explanation:
From the graph, we can conclude that,
1. The two lines are continuous lines and not broken lines. So, the inequality sign should be either
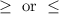 .
.
2. The points on the lines of the shaded region are also included in the solution.
The only option that matches with the above conditions is option D. So, option D is the correct answer.
Let us verify it.
Now, let us consider a point that is inside the shaded region and also on any one line. Let us take
 .
.
Plug in 0 for x and 2 for y in each of the options and check which inequality holds true.
Option A:
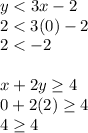
So, inequality 1 is wrong as -2 is less than 2.
Option B:
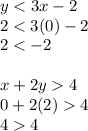
Both the inequalities are wrong.
Option C:
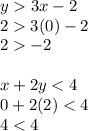
Inequality 2 is wrong.
Option D:
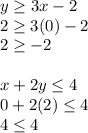
Here, both inequalities are correct.
So, option D is the correct answer.