Question

(a) A gambler has a fair coin and a two-headed coin in his pocket. He selects one of the the coins at random; when he flips it, it shows heads. What is the probability that it is the fair coin? (b) Suppose that he flips the same coin a second time and, again, it shows heads. Now what is the probability that it is the fair coin? (c) Suppose that he flips the same coin a third time and it shows tails. Now what is the probability that it is the fair coin?

Answer 1

a)
`(1)/(3)` ≈0.33

b)
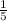`(1)/(5)` = 0.2

c) 1

Explanation:

This is a conditional probability problem.

Let P(F)= the probability that the gambler selects fair coin

P(TH) = the probability that the gambler selects two head coin

P(H|TH)= the probability of getting heads given that the gambler selects two headed coin.

P(H|F)= probability of getting heads given that the gambler selects fair coin

P(F|H)= probability of the gambler selects fair coin given that it shows head after flipping

P(H)= the probability of getting heads

P(HH)=the probability of getting two heads

Since gambler selects coins randomly P(F)=P(TH)= 0.5, P(H|F)=0.5 since the coin is fair.

We have also this conditional probability equation:

P(F|H) =
`(P(F)*P(H|F))/(P(H))`

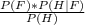
a) For the first case P(H)= P(H|F)×P(F)+P(H|TH)×P(TH)=
`(1)/(2)`×
`(1)/(2)`+1×
`(1)/(2)`=
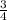`(3)/(4)` =0.75

If we put the numbers in conditional probability formula:

P(F|H) =
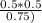`(0.5*0.5)/(0.75))` =
`(1)/(3)`≈0.33

b) For flipping twice:

P(HH)= P(HH|F)×P(F)+P(HH|TH)×P(TH) =
`(1)/(4)`×
`(1)/(2)`+1×
`(1)/(2)`=
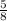`(5)/(8)` =0.625

then our formula becomes:

P(F|HH) =
`(0.5*0.25)/(0.625))` =
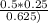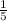`(1)/(5)` =0.2

c) For flipping third time:

When flipping for the third time and it shows tails, then the coin cannot be two heads coin since it has 0 probability of getting tails. Therefore for this case the P(F|T)= the probability of choosing fair coin given that it shows tails)=1