Question

The weights for newborn babies is approximately normally distributed with a mean of 6.2 pounds and a standard deviation of 2 pounds. Consider a group of 1400 newborn babies: 1. How many would you expect to weigh between 5 and 8 pounds? 2. How many would you expect to weigh less than 7 pounds? 3. How many would you expect to weigh more than 6 pounds? 4. How many would you expect to weigh between 6.2 and 9 pounds?

Answer 1

1. 758 newborn babies

2. 918 newborn babies

3. 756 newborn babies

4. 587 newborn babies

Explanation:

Let's start by defining the following event :

W : ''The weight of a newborn baby''

W ~ N(μ,σ) Where N is the normal distribution, μ is the mean and σ is the standard deviation

W ~ N(6.2,2)

To calculate probability, we need to turn this variable into a N(0,1) by doing the following :

First we subtract the mean to W and then we divide by the standard deviation

[(W-μ) / σ] ~ N(0,1)

1.
`P(5<W<8)`

`P((5-(6.2))/(2)<(W-(6.2))/(2)<(8-(6.2))/(2))=`

`P(-0.6<Z<0.9)`

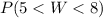
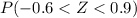
Where Z ~ N(0,1)

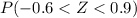`P(-0.6<Z<0.9)` is the area below the normal curve N(0,1) between the values -0.6 and 0.9

P(-0.6<Z<0.9) = Φ(0.9) - Φ(-0.6)

Where Φ is the cumulative distribution for N(0,1)

P(-0.6<Z<0.9) = Φ(0.9) - Φ(-0.6) = 0.8159 - 0.2743 = 0.5416

Then the probability of the variable W to be between 5 and 8 pounds is 0.5416

To find the number of newborn babies expected to weigh between 5 and 8 pounds we multiply the group of 1400 and the probability

`(1400).(0.5416)=758.24`

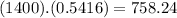
758.24 ≅ 758

Then 758 newborn babies are expected to weigh between 5 and 8 pounds

2.

`P(W<7)=P(Z<(7-6.2)/(2))=P(Z<0.4)`

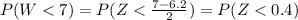
P(Z<0.4) = Φ(0.4) = 0.6554

The expected number of newborn babies is

`(1400).(0.6554)=917.56`

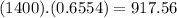
917.56 ≅ 918

918 newborn babies are expected to weigh less than 7 pounds

3.

`P(W>6)=1-P(W<6)=1-P(Z<(6-6.2)/(2))=1-P(Z<-0.1)`

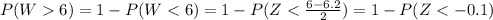
1-P(Z<-0.1) = 1 - Φ(-0.1) = 1 - 0.4602 = 0.5398

The expected number of babies is

`(1400).(0.5398)=755.72`

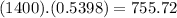
755.72 ≅ 756

The expected number of babies to weigh more than 6 pounds is 756

4.

`P(6.2<W<9)=P((6.2-6.2)/(2)<Z<(9-6.2)/(2))=P(0<Z<1.4)`

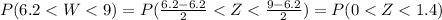
P(0<Z<1.4) = Φ(1.4) - Φ(0) = 0.9192 - 0.500 = 0.4192

The expected number of babies is

`(1400).(0.4192)= 586.88`

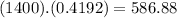
586.88 ≅ 587

587 newborn babies are expected to weigh between 6.2 and 9 pounds