Answer:
The best score is 200 points, answering 20 items of type A.
Explanation:
Let the following variables be:
x: items of type A
y: items of type B
The first condition is that it takes 3 minutes for each item of type A and 6 minutes for an item of type B. Total time allowed is 60 minutes. The equation would be:
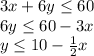
Furthermore, you may answer more than 16 questions. The second equation would be:
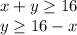
We show the plots of both equations in the attached pictures we find 3 critical points:
P₁ (16,0); P₂ (12,4); P₃ (20,0)
The score of the test would be:
S= 10x + 15y
We evaluate the points
P₁ (16,0)
S(16,0)= 10×16 + 15×0=160 points
P₂ (12,4)
S(16,0)= 10×12 + 15×4=180 points
P₃ (20,0)
S(20,0)= 10×20+ 15×0=200 points