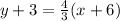Question

Complete the equation of the line through (-9,-7) and (-6,-3)

Answer 1

The equation of the line through (-9, -7) and (-6, -3) is
`y+3=(4)/(3)(x+6)`

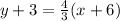
Solution:

Given, two points are (-9, -7) and (-6, -3)

We have to find that a line that passes through the given two points.

First let us find the slope of the line that passes through given two points.

The slope of the line "m" is given as:

`\mathrm{m}=(y_(2)-y_(1))/(x_(2)-x_(1))`

`\text { where, }\left(x_(1), y_(1)\right) \text { and }\left(x_(2), y_(2)\right) \text { are two points on line. }`

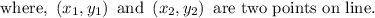
So slope of our line
`=(-3-(-7))/(-6-(-9))=(-3+7)/(-6+9)=(4)/(3)`

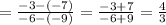
Now, let us find the line equation using point slope form:

`\mathrm{y}-\mathrm{y}_(1)=\mathrm{m}\left(\mathrm{x}-\mathrm{x}_(1)\right) \text { where } \mathrm{m} \text { is slope and }\left(\mathrm{x}_(1), \mathrm{y}_(1)\right) \text { is point on the line. }`

By substituting the values we get,

`\text { Then, line equation } \rightarrow y-(-3)=(4)/(3)(x-(-6))`

`y+3=(4)/(3)(x+6)`

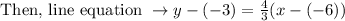
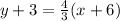
Hence, the line equation is
`y+3=(4)/(3)(x+6)`