Question

On comparing the ratios of the coefficients, find out whether the pair of equations x – 2y =0 and 3x + 4y -20 =0 is consistent or inconsistent.​

Answer 1

The pair of equations is consistent

Explanation:

A consistent system of equations has at least one solution

• The consistent independent system has exactly 1 solution

• The consistent dependent system has infinitely many solutions

An inconsistent system has no solution

In the system of equations ax + by = c and dx + ey = f, if

1. a = d, b = e, and c = f, then the system is consistent dependent and has infinitely many solutions
2. a = d, b = e, and c ≠ f, then the system is inconsistent and has no solution
3. a ≠ d, and/or b ≠ e, and/or c ≠ f, and
   `(a)/(d)` ≠
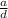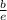   `(b)/(e)` , then the system is consistent independent and has exactly one solution

∵ x - 2y = 0

∴ The coefficient of x ⇒ a = 1

∴ The coefficient of y ⇒ b = -2

∴ The numerical term ⇒ c = 0

∵ 3x + 4y - 20 = 0

→ Add 20 to both sides

∴ 3x + 4y - 20 + 20 = 0 + 20

∴ 3x + 4y = 20

∵ The coefficient of x ⇒ d = 3

∵ The coefficient of y ⇒ e = 4

∵ The numerical term ⇒ f = 20

∵ a ≠ d

∵ b ≠ e

∵ c ≠ f

∵
`(a)/(d)` =
`(1)/(3)`

∵
`(b)/(e)` =
`(-2)/(4)` =
`(-1)/(2)`

∴
`(a)/(d)` ≠
`(b)/(e)`

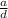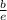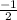
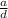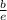
→ By using rule 3 above

∴ The pair of equations is consistent