Answer:
Simplified form of fifth root of x times the fifth root of x times the fifth root of x times the fifth root of x is
![\sqrt[5]{x^(4)}](https://img.qammunity.org/2020/formulas/mathematics/middle-school/2xty7qaur7rzu9fv0gu6ld7pm06v7hxwob.png)
Solution:
Need to find simplified form of fifth root of x times the fifth root of x times the fifth root of x times the fifth root of x. That is need to find simplified form of following expression.
![\sqrt[5]{x} * \sqrt[5]{x} * \sqrt[5]{x} * \sqrt[5]{x}](https://img.qammunity.org/2020/formulas/mathematics/middle-school/4bofkrwkhdbcbwk0g5xh4737lxg2uf1jf6.png)
![\text { since } \sqrt[n]{a}=(a)^{(1)/(n)}](https://img.qammunity.org/2020/formulas/mathematics/middle-school/z9jkzkz9xc7c9k6jnnek7gjnbr53n3t07p.png) we get
we get
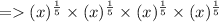
Now using law of exponent that is
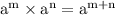
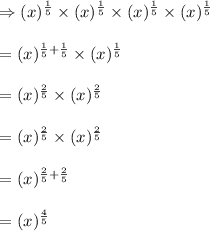
Using another law of exponent that is
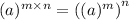 we get
we get
![\begin{array}{l}{(x)^{(4)/(5)}=(x)^{4 * (1)/(5)}=\left((x)^(4)\right)^{(1)/(5)}} \\\\ {\left((x)^(4)\right)^{(1)/(5)}=\sqrt[5]{x^(4)}}\end{array}](https://img.qammunity.org/2020/formulas/mathematics/middle-school/s907pwldijysvxqbiiua3s50bo5ej33zwp.png)
Hence the simplified form of fifth root of x times the fifth root of x times the fifth root of x times the fifth root of x is
![\sqrt[5]{x^(4)}](https://img.qammunity.org/2020/formulas/mathematics/middle-school/2xty7qaur7rzu9fv0gu6ld7pm06v7hxwob.png)