Answer:
Option C = 15
Explanation:
In principle when a function f(x) varies directly with x it suggests that any changes in x results in the equivalent changes in f(x). If we have two variables, i.e. y representing f(x) and x representing itself, any increment/decrement in x will result to the same increment/decrement in y by a factor a, thus we can say that y = ax, implying y and x have the same ratio.
In the given question we know that
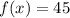 when
when
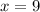 , which translates as
, which translates as

This tells us that
 varies by a factor (lets call it)
varies by a factor (lets call it)
 for a given value of
for a given value of
 .
.
To find this factor we can just divide 45 with 9 which gives:
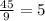
Thus the factor
 here is
here is
 which finally tells us that
which finally tells us that
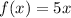 Eqn (1) our original function.
Eqn (1) our original function.
Since we now know our function we can plug in the value for
 and solve for
and solve for
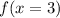 as follow:
as follow:
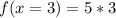

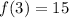
Looking at the given options in the question we can conclude that the correct answer is Option C = 15