Question

Find AA and BB that make the equation true. Verify your results.

a. A / x + 1 +B / x − 1 = 2 / x2 − 1
b.A / x + 3 + B / x + 2 = 2x − 1 / x2 + 5x + 6

Answer 1

a. A = -1 and B = 1

b. A = 7 and B = -5

Explanation:

a.

`(A)/(x+1) +(B)/(x-1)  = (2)/(x^2-1)`

`(A*(x-1)+B*(x+1))/((x+1)*(x-1)) = (2)/(x^2-1)`

`(Ax - A + Bx + B)/(x^2 -1) = (2)/(x^2-1)`

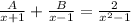
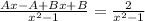
To the equation be true, the "x-parts" and "nonx-parts" mist be the same, so:

Ax + Bx = 0

(A + B)x = 0

A + B = 0

A = -B

B - A = 2

B - (-B) = 2

2B = 2

B = 1 and A = -1

b.

`(A)/(x+3) + (B)/(x +2) = (2x -1)/(x^2+5x+6)`

`(A*(x+2) + B*(x+3))/((x+3)*(x+2)) = (2x-1)/(x^2+5x+6)`

`(Ax + 2A + Bx + 3B)/(x^2 + 5x + 6) = (2x-1)/(x^2+5x+6)`

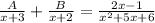
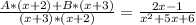
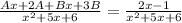
To the equation be true, the "x-parts" and "nonx-parts" mist be the same, so:

Ax + Bx = 2x

(A + B)x = 2x

A + B = 2

A = 2 - B

2A + 3B = -1

2*(2-B) + 3B = -1

4 - 2B + 3B = -1

B = -5 and A = 2 - (-5) = 7