Answer:
7 hours
Explanation:
Since, the final amount of caffeine formula would be,
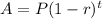
Where,
P = Initial value,
r = rate per period,
t = number of periods,
Here, r = 10% = 0.10, t = h, A =
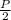
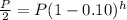
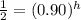
Taking log both sides,
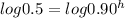
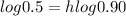
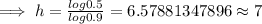
Hence, after 7 hours the amount of caffeine in a body to reduce by half.