Answer:
The zeros of the function f(x) = 9x^3 - 45x^2 + 36x is 0, 1, 4
Solution:
Given that

For finding the zeros of the function, we equate the entire function to zero i.e.,
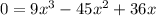
Dividing throughout by 9, we get

Taking x as common throughout the equation, we get
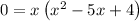
Thus, by factorization of the above equation, we get 0 = x(x - 1)(x - 4)
Now ,equating the factors we got to 0, we get
x = 0, x - 1 = 0, x - 4 = 0
x = 0, x = 1, x = 4
Thus, the zeros of the above given function are 0, 1, 4