Answer:

Explanation:
Tangent to y-axis means that the side of the circle TOUCHES the y axis.
Since the center is at (-7,2) and it touches the y axis, we can figure out the radius. It goes from (-7,2) to y-axis. Horizontally, the center is 7 units left of y-axis, so that is the radius ----- 7 units
The standard form of a circle is:

Where
(h,k) is the center
r is the radius
Putting the information into the form, we have:
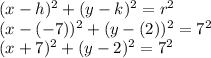
THis is the standard form.