Question

In triangle MNP if the measure of angle R is five more than twice x, and the measure of angle S is one more than x, and the measure of angle T is sixteen less than seven times x, whats x

Answer 1

x = 19

Explanation:

Let's convert the given statements into algebraic expressions.

R is five more than twice x:

R = 2x + 5

S is one more than x:

S = x + 1

T is sixteen less than seven times x:

T = 7x - 16

Also, we know, the sum of 3 angles in a triangle is ALWAYS EQUAL to 180 degrees. Thus we can write:

R + S + T = 180

Substituting the expressions, we have:

2x + 5 + x + 1 + 7x - 16 = 180

Now we do algebra and simply solve for x. Shown below:

`2x + 5 + x + 1 + 7x - 16 = 180\\10x-10=180\\10x=180+10\\10x=190\\x=(190)/(10)\\x=19`

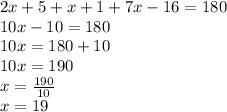
Hence, x = 19