Question

14.

For Exercises 7 through 25, assume that the sample is taken
from a large population and the correction factor can be
ignored. on no 2000 o algo
7. Life of Smoke Detectors The average lifetime of smoke
detectors that a company manufactures is 5 years, or 60
months, and the standard deviation is 8 months. Find the
probability that a random sample of 30 smoke detectors
will have a mean lifetime between 58 and 63 months.

Answer 1

0.8945

Explanation:

The average life of smokers,
`\mu` is 60 months

Standard deviation is 8 months

Random sample, n is 30 smoke detectors

`P(58 < \bar x < 63)`

=
`P((58 - \mu)/(\sigma/\sqrt n) < (\bar x - \mu)/(\sigma/\sqrt n) < (63 - \mu)/(\sigma/\sqrt n))`

=
`P((58 - 60)/(8/\sqrt 30) < Z < (63 - 60)/(8/\sqrt 30))`

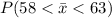
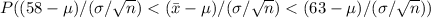
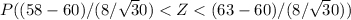
= P(-1.37 < Z < 2.05)

= P(Z < 2.05) - P(Z < -1.37) Then from the standard tables, the Z values are 0.9798 for Z=2.05 and 0.0853 for Z=-1.37

= 0.9798 - 0.0853

= 0.8945

Therefore, the probability that a random sample of 30 smoke detectors will have a lifetime between 58 months and 63 months is 0.8945