Answer:
58.0 days
Explanation:
The equation for the radioactive decay is
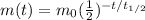
where
m(t) is the mass of the sample left at time t
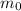 is the initial mass of the sample
is the initial mass of the sample
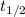 is the half-life
is the half-life
For the phosporus-32 isotope in the problem, we have:
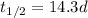 (half-life in days)
(half-life in days)
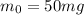 is the initial mass
is the initial mass
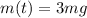 is the mass at time t
is the mass at time t
Solving for t, we find the time needed for the sample to reduce to 3 mg:
