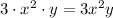Answer:
C.
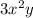
Explanation:
Given the trinomial

consisting of three terms.
Find the greatest common factor of numbers:
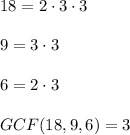
Find the greatest common factor of powers x:
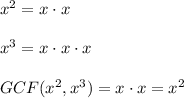
Find the greatest common factor of powers y:

Thus, the greatest common factor of the whole terms is