Question

:) The cost price of 11 pencils is equal to the selling price of 10 pencils. Find the gain

or loss per cent.​

Answer 1

You are losing 9 percent for every cent or 0.09cents per cent.

Explanation:

Answer 2

Explanation:

❅
`\underline{ \underline{ \text{Given}}} :`

• CP of 11 pencils = SP of 10 pencils

❅
`\underline{ \underline{ \text{To \: find}}}:`

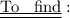
• Gain percent or loss percent

✻
`\underline{ \underline{ \text{Solution}}} :`

`\sf{CP\: of \: 1 \: pencil = x \: ( \: suppose})`

`\sf{CP \: of \: 11 \: pencils = 11x}`

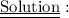
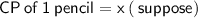
`\sf{SP \: of \: 10 \: pencils = 11x}` { Since CP of 15 articles is equal to SP of 12 )

`\sf{SP \: of \: 1 \: pencil = (11)/(10) x}`

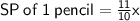
Here , SP > CP. So, He / she made a profit.

`\boxed{ \sf{Profit = SP - CP}}`

⟿
`\sf{ (11)/(10) x - x}`

⟿
`\sf{ (11x - 10x)/(10)}`

⟿
`\sf{ (x)/(10) }`

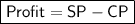
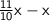
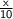
Now , Finding the profit percentage :

`\boxed{ \sf{Profit\% \: = (Profit)/(CP) * 100\%}}`

⟿
`\sf{ ( (x)/(10) )/(x) } * 100\%`

⟿
`\sf{ (x)/(10) * (1)/(x) * 100\%}`

⟿
`\sf{ \frac{ \cancel{x}}{10} * \frac{1}{ \cancel{x} } * 100\%}`

⟿
`\sf{ (1)/(10) * 100\%}`

⟿
`\boxed{ \sf{10\%}}`

`\purple{ \boxed{ \boxed{ \tt{⇢Our \: final \: answer : 10\% \: \: profit}}}}`

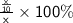
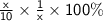
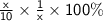
Hope I helped ! ♡

Have a wonderful day / night ツ

▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁