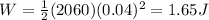Question

When a 4.20-kg object is hung vertically on a certain light spring that obeys Hooke's law, the spring stretches 2.00 cm. (a) If the 4.20-kg object is removed, how far will the spring stretch if a 1.50-kg block is hung on it? 0.714 Correct: Your answer is correct. cm (b) How much work must an external agent do to stretch the same spring 4.00 cm from its unstretched position? 3.02 Incorrect: Your answer is incorrect. J

Answer 1

(a) 0.714 cm

First of all, we need to find the spring constant of the spring. This can be done by using Hooke's law:

`F=kx`

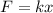
where

F is the force applied on the spring

k is the spring constant

x is the stretching of the spring

At the beginning, the force applied is the weight of the block of m = 4.20 kg hanging on the spring, therefore:

`F=mg=(4.20)(9.8)=41.2 N`

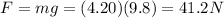
The stretching of the spring due to this force is

x = 2.00 cm = 0.02 m

Therefore, the spring constant is

`k=(F)/(x)=(41.2)/(0.02)=2060 N/m`

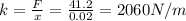
Now, a new object of 1.50 kg is hanging on the spring instead of the previous one. So, the weight of this object is

`F=mg=(1.50)(9.8)=14.7 N`

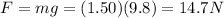
And so, the stretching of th spring in this case is

`x=(F)/(k)=(14.7)/(2060)=0.00714 m = 0.714 cm`

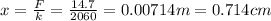
(b) 1.65 J

The work done on a spring is given by:

`W=(1)/(2)kx^2`

where

k is the spring constant

x is the stretching of the spring

In this situation,

k = 2060 N/m

x = 4.00 cm = 0.04 m is the stretching due to the external agent

So, the work done is

`W=(1)/(2)(2060)(0.04)^2=1.65 J`