Answer:
0.95 atm
Step-by-step explanation:
We are given;
Initial pressure, P1 = 1.0 atm
Initial temperature, T1 =298 K (25°C + 273)
Initial volume, V1 = 0.985 L
Final temperature, T2 = 295 K (22°C + 273)
Final volume, V2 = 1.030 L
We are required to find final air pressure;
Using the combined gas law;
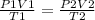
To get, P2 ;
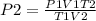
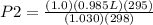
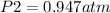
= 0.95 atm
Therefore, the air pressure at the top of the mountain is 0.95 atm