Question

You toss a 0.40-kg ball at 9.0 ms/ to a 14-kg dog standing on an iced-over pond. The dog catches the ball and begins to slide on the ice. (a) Measured from the Earth reference frame, what is the velocity of the dog immediately after he catches the ball? (b) Measured from the Earth reference frame, what is the velocity of an inertial reference frame in which the ball’s kinetic energy does not change? (c) Measured from the Earth reference frame, how much of the original kinetic energy of the system is convertible? (d) Measured from the reference frame described in part b, how much of the original kinetic energy of the system is convertible?

Answer 1

a)
`v_(f)` = 0.25 m / s b) u = 0.25 m / s

Step-by-step explanation:

a) To solve this problem let's start with the conservation of the moment, for this we define a system formed by the ball plus the dog, in this case all the forces are internal and the moment is conserved

We will write the data

m₁ = 0.40 kg

v₁₀ = 9.0 m / s

m₂ = 14 kg

v₂₀ = 0

Initial

po = m₁ v₁₀

Final

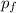`p_(f)` = (m₁ + m₂) vf

po = pf

m₁ v₁₀ = (m₁ + m₂)
`v_(f)`

`v_(f)` = v₁₀ m₁ / (m₁ + m₂)

`v_(f)` = 9.0 (0.40 / (0.40 +14)

`v_(f)` = 0.25 m / s

b) This is the reference frame of the center of mass of the system in this case the speed of this frame is the speed of the center of mass

u = 0.25 m / s

In the direction of movement of the ball

c) Let's calculate the kinetic energy in both moments

Initial

K₀ = ½ m₁ v₁₀² +0

K₀ = ½ 0.40 9 2

K₀ = 16.2 J

Final

`K_(f)`= ½ (m₁ + m₂)
`v_(f)`2

`K_(f)` = ½ (0.4 +14) 0.25 2

`K_(f)` = 0.45 J

ΔK = K₀ -
`K_(f)`

ΔK = 16.2-0.445

ΔK = 1575 J

These will transform internal system energy

d) In order to find the kinetic energy, we must first find the velocities of the individual in this reference system.

v₁₀’= v₁₀ -u

v₁₀’= 9 -.025

v₁₀‘= 8.75 m / s

v₂₀ ‘= v₂₀ -u

v₂₀‘= - 0.25 m / s

`v_(f)` ‘=
`v_(f)` - u

`v_(f)` = 0

Initial

K₀ = ½ m₁ v₁₀‘² + ½ m₂ v₂₀‘²

Ko = ½ 0.4 8.75² + ½ 14.0 0.25²

Ko = 15.31 + 0.4375

K o = 15.75 J

Final

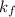`k_(f)` = ½ (m₁ + m₂) vf’²

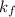`k_(f)` = 0

All initial kinetic energy is transformed into internal energy in this reference system